Answer:
We need a sample size of at least 657.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.
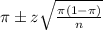
In which
z is the zscore that has a pvalue of
 .
.
The margin of error is given by:
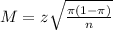
45 percent of its claims have errors.
So
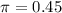
99% confidence level
So
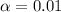 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
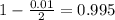 , so
, so
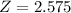 .
.
What sample size is needed if they wish to be within 5 percent of the actual
This is a sample size of at least n, in which n is found when M = 0.05.
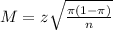
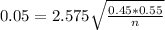

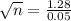
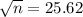
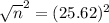
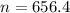
We need a sample size of at least 657.