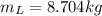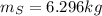Question

6. Two blocks with different masses are attached to either end of a light rope that passes over a light, frictionless pulley suspended from the ceiling. The masses are released from rest, and the more massive one starts to descend. After this block has descended 1.20 m, its speed is 3.00 m/s. If the total mass of the two blocks is 15.0 kg, what is the mass of each block

Answer 1

The mass of the blocks are 8.704 kg and 6.296 kg.

Step-by-step explanation:

Let's first find the acceleration the causes this change in speed:

`V^2 - U^2 = 2*a*s`

`3^2 - 0^2 = 2 * a * 1.2`

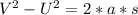
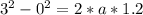
a = 3.75 m/s^2

We can now write the following equation:

`F = m_s * a`

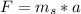
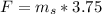`F = m_s * 3.75` -Equation 1

Here the mass taken is of the small block. The force will be the difference in weight of the two blocks (
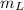`m_L` is the larger block and
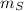`m_S` is the smaller block).

So we can write two more equations:

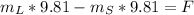`m_L*9.81 - m_S*9.81 = F` -Equation 2

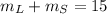`m_L+m_S=15` -Equation 3

Solving the three equations simultaneously we get:

F = 23.612 N

`m_L=8.704 kg`

`m_S= 6.296 kg`