Question

In a study of the accuracy of fast food​ drive-through orders, one restaurant had 36 orders that were not accurate among 322 orders observed. Use a 0.01 significance level to test the claim that the rate of inaccurate orders is equal to​ 10%. Does the accuracy rate appear to be​ acceptable?

Answer 1

Explanation:

Given that in a study of the accuracy of fast food​ drive-through orders, one restaurant had 36 orders that were not accurate among 322 orders observed.

Sample proportion = 36/322= 0.112

Hypotheses would be:

`H_0:p=0.10\\H_a: p\\eq 0.10`

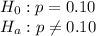
(two tailed test at 1% significance level)

p difference = 0.012

Std error =
`\sqrt{(0.1*0.9)/(322) } \\=0.017`

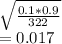
Test statistic =
`(0.012)/(0.017) \\=0.706`

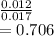
p value = 0.48

since p >0.01 we accept null hypothesis

The accuracy rate appears to be acceptable as only 10% are not accurate.