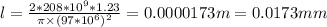Answer:
0.0173 mm
Step-by-step explanation:
The maximum length of eliptical flow will be given by
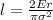 where l is the maximum length of elliptical flow, E is the modulus of elasticity, r is the surface energy of magnesium oxide and nickel,
where l is the maximum length of elliptical flow, E is the modulus of elasticity, r is the surface energy of magnesium oxide and nickel,
 is the tensile stress.
is the tensile stress.
Substituting 208 Gpa for E, 1.23 for r and 97 Mpa for tensile stress then