Question

An article reported that for a sample of 48 kitchens with gas cooking appliances monitored during a one-week period, the sample mean CO2 level (ppm) was 654.16, and the sample standard deviation was 166.25. (a) Calculate and interpret a 95% (two-sided) confidence interval for true average CO2 level in the population of all homes from which the sample was selected. (Round your answers to two decimal places.)

Answer 1

The 95% confidence interval for true average CO2 level in the population of all homes from which the sample was selected is between 607.13 ppm and 701.19 ppm. This means that we are 95% sure that the true average CO2 level in the population of all homes from which the sample was selected is between 607.13 ppm and 701.19 ppm.

Explanation:

We have that to find our
`\alpha` level, that is the subtraction of 1 by the confidence interval divided by 2. So:

`\alpha = (1-0.95)/(2) = 0.025`

Now, we have to find z in the Ztable as such z has a pvalue of
`1-\alpha`.

So it is z with a pvalue of
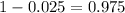`1-0.025 = 0.975`, so
`z = 1.96`

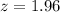
Now, find M as such

`M = z*(\sigma)/(√(n))`

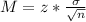
In which
`\sigma` is the standard deviation of the population and n is the size of the sample.

`M = 1.96*(166.25)/(√(48)) = 47.03`

The lower end of the interval is the sample mean subtracted by M. So it is 654.16 - 47.03 = 607.13

The upper end of the interval is the sample mean added to M. So it is 654.16 + 47.03 = 701.19

The 95% confidence interval for true average CO2 level in the population of all homes from which the sample was selected is between 607.13 ppm and 701.19 ppm. This means that we are 95% sure that the true average CO2 level in the population of all homes from which the sample was selected is between 607.13 ppm and 701.19 ppm.