Question

Choose the graphic representation of the quadratic function f(x) = x2 - 8x + 24.

Answer 1

Answer: f(x) = (x-4)^2+8 for vertex form.

Explanation:

Given Quadratic Function f(x) = x^2-8x+24

As you know, the standard form is y = ax^2+bx+c where a,b and c are part of real numbers. and a musn't be 0

For vertex form, a(x-h)^2+k and (h,k) is vertex of graph.

We are going to change the standard form to vertex form so we can graph it easily.

`f(x)=x^2-8x+24`

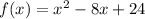
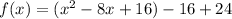`f(x)=(x^2-8x+16)-16+24` By completing the square.

`f(x)=(x-4)^2+8`

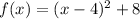
Now we know vertex and that is (4,8)

For Axis of Symmetry = h

Then draw the graph.

From the graph, vertex is not on x-axis, meaning that there are no common points (The equation has a complex solution/answer.)