Answer:
For t=3 sec the velocity change from positive to negative
Explanation:
we have
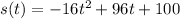
This is the equation of a vertical parabola open downward (the leading coefficient is negative)
where
s(t) is the distance in feet
t is the time in seconds
We know that
To find out when the velocity change from positive to negative, we need to determine the turning point of the quadratic equation
The turning point of the quadratic equation is the vertex
so
Convert the quadratic equation into vertex form
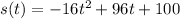
Factor -16
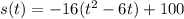
Complete the square
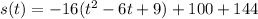
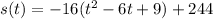
Rewrite as perfect squares
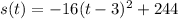
The vertex is the point (3,244)
therefore
For t=3 sec the velocity change from positive to negative