Question

An ideal parallel-plate capacitor consists of a set of two parallel plates of area AAA separated by a very small distance ddd. When this capacitor is connected to a battery that maintains a constant potential difference between the plates, the energy stored in the capacitor is UUU0. If the separation between the plates is doubled, how much energy is stored in the capacitor?

Answer 1

Hi your question lacks the options here is the complete question

An ideal parallel-plate capacitor consists of a set of two parallel plates of area AAA separated by a very small distance ddd. When this capacitor is connected to a battery that maintains a constant potential difference between the plates, the energy stored in the capacitor is UUU0. If the separation between the plates is doubled, how much energy is stored in the capacitor?

a)2U0

b)U0/2

c)4U0

d)U0/4

e)U0

Answer :
`(U0)/(2)` ( b )

Step-by-step explanation:

Firstly we calculate the capacitance of a parallel plate capacitor

C =
`(e A)/(d)`

where e = permittivity of free space,

d = distance between plates

A = Area of plates

Note: for a parallel plate capacitor when the distance between the plates is increased by a factor of 2 ( doubled ) the capacitance of the capacitor is decreased by factor of 2

hence the new capacitance would be

C₂ =
`(C)/(2)`

secondly we calculate the energy stored in a parallel plate capacitor

U₀ =
`(CV^(2) )/(2)` ( equation 1 )

where c = capacitance of the capacitor

V = voltage

Note: for a parallel plate capacitor when the capacitance is reduced by a factor of 2 the energy stored in the capacitor also decreases by a factor of 2 as well

hence the new energy stored would be

U₂ =
`(1)/(2)` * (
`(CV^(2) )/(2)`) (equation 2 )

substitute
`(CV^(2) )/(2)` with U₀ in equation 2

The new energy becomes

U₂ =
`(1)/(2)` * U =
`(U0)/(2)`

Answer 2

When the distance is doubled the energy stored would reduce by half

So the energy stored would be half of the original energy

Step-by-step explanation:

Here we are looking for how the potential energy stored on a capacitor changes as the the distance between the plates is being double at a constant voltage

We are going to be making use of two equations

First One is

`U = (1)/(2) C V^2`

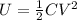
Where u is the potential energy

C is the capacitance of the capacitor

V is the voltage

Second one is

`C = (\epsilon_0A)/(d)`

Where
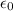`\epsilon_0` defines how well electricity travels through free space

A is the area

d is the distance between the two plates

Looking a the capacitance

The initial capacitance
`C_0 = (\epsilon_0A)/(d_0)`
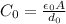
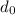`d_0` is the original distance

Now the new capacitance

`C_(new) = (\epsilon_0A)/(d_(new))`

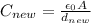
From the question

`d_(new) = 2 d_(0)`

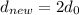
Therefore the new capacitance

`C_(new) = (\epsilon_0A)/(2d_(0))`

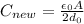
Hence looking a the above equation we see that

`C_(new) = (1)/(2) C_0`

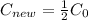
Looking a the potential energy

The original potential energy
`U_0 = (1)/(2) C_0 V^2`

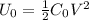
The new potential energy
`U_(new) = (1)/(2) C_(new) V^2`

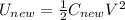
Substituting
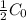`(1)/(2) C_0` for
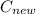`C_(new)` in the equation above

`U_(new) = (1)/(2) ((1)/(2) C_0)V^2`

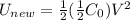
looking a this equation we see that
`(1)/(2) C_0 V^2 \ is \ equal \ to \ U_0` So

`U_(new) =(1)/(2) U_0`

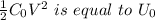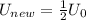
So When the distance is doubled the energy stored would reduce by half