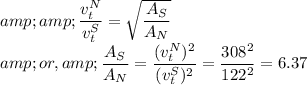Question

The terminal speed of a sky diver is 122 km/h in the spread-eagle position and 308 km/h in the nosedive position. Assuming that the diver's drag coefficient C does not change from one position to the other, find the ratio of the effective cross-sectional area A in the slower position to that in the faster position.

Answer 1

The ratio of the cross-sectional area inthe slower position to that in the faster position is
`\bf{6.37}`

Step-by-step explanation:

The terminal velocity of any object is given by

`v_(t) = \sqrt{(2 mg)/(C \rho A)}`

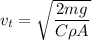
where '
`m`' is the mass of the object, '
`g`' is the acceleration due to gravity, '
`C`' is the drag coefficient, '
`\rho`' is the density and '
`A`' is the cross-sectional area of the object.

For spread-eagle position if
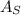`A_(S)` be the cross-sectional area and for nosedive position if
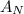`A_(N)` be the cross-sectional area, then from the above expression

`&& v_(t)^(S) = \sqrt{(2 mg)/(C \rho A_(S))}\\&and,& V_(t)^(N) = \sqrt{(2 mg)/(C \rho A_(N))}`

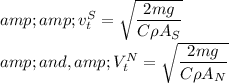
where
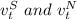`v_(t)^(S)~and~v_(t)^(N)`are the terminal velocities for spread-eagle position and nosedive position respectively.

Taking the ratio of both the velocities,

`&& (v_(t)^(N))/(v_(t)^(S)) = \sqrt{(A_(S))/(A_(N))}\\&or,& (A_(S))/(A_(N)) = ((v_(t)^(N))^(2))/((v_(t)^(S))^(2)) = (308^(2))/(122^(2)) = 6.37`