Question

A thermocouple place in the mouth of a pitot tube can be used to measure the stagnation temperature of the flow. Such an arrangement placed in an air flow gives the stagnation pressure as 180 kPa, the static pressure as 55 kPa and stagnation temperature as 95

Answer 1

velocity of stream =
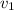`v_(1)` = 461m/s

Step-by-step explanation:

see stagnation temperature = 95 C = 95 + 273 = 368 K

now stagnation pressure = P1 = 180 pa

and P2 55 Kpa

now for air specific heta ratio = y = 1.4

for supersonic flow : T2/T1 = (P2/P1)
`(y-1)/y{}`

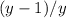
so, T2/368 = (55/180)
`{(1.4-1)/1.4}`

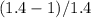
so T2 = 262.26 K = static Temperature

now, if we apply energy balance equation b/w static and stagnation point :

we get :

Cp.T1 + v1^2/2 = Cp.T2 + V2^2/2

here Cp = 1.005 KJ/Kg.K

and V2 = 0 as at static point velocity = 0

so, 1.005 x 368 + V1^2/2 x 1000 = 1.005 x 262.6

so,velocity of stream = V1 = 461m/s