Question

Consider the number 378, 000. (a) Count the number of positive integral factors. (b) Of these factors, how many are even? (c) How many are divisible by both 3 and 7? (d) How many zeros are at the end of 378, 000!

Answer 1

Explanation:

A) 2^3 * 3^3 * 5 ^ 2 * 7 ^ 1

Total factor of 378,000 including itself and 1 is

(3+1)*(3+1)*(2+1)*(1+1) = 4*4*3*2 = 96

B) Knowing its prime factorization we can solve this problem

378,000 = 378* 100

= 2 *27*7*10*10

= 2*3*3*3*7*5*2*5*2

All the above are indivisible by any number except itself and one, or are now prime numbers,from here we regroup

= 2*2*2*3*3*3*5*5*7

This can be given as 2^3 * 3^3 * 5 ^ 2 * 7 ^ 1

making the x= 0 for 2 ^ x

it becomes 2^0 * 3 ^ 3 * 5 ^ 2 * 7 ^ 1

We want the numbers of factors of the above

Therefore (0 + 1 )( 3+1)(2+1)(1+1) = 1 *4*3*2 = 24

C) From answer (B) 2^0 * 3 ^ 3 * 5 ^ 2 * 7 ^ 1 Is a factor of 378,000

And from it three the power of three and 7 are been added to know the exact time 3 and 7 can successfully divide 378,000

3+1 = 4

D) Trailing zeros are a sequence of 0's in the decimal representation (or more generally, in any positional representation) of a number, after which no other digits follow

378,000 Have three trailing zeros

Number of trailing zeros in the decimal representation of n!, the factorial of a non-negative integer n, can be determined with this formula

`(n)/(5^(2) )`+

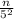Solution:

378,000!

`(378,000)/(5)` +
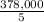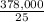`(378,000)/(25)` = 75,600 + 15,120 = 90,720 trailing zeros