Question

A fourth grade class of 28 students is given a standardized math test. The mean score of the 12 boys is 25 with a standard deviation of 3. The mean score of the 16 girls is 24 with a standard deviation of 4. What is the standard error for the sampling distribution

Answer 1

The standard error for the sampling distribution is 1.323.

Explanation:

Let X = scores of girls and Y = scores of boys.

The information provided is:

`\bar x=24\\s_(x)=4\\n_(x)=16\\\bar y=25\\s_(y)=3\\n_(y)=12`

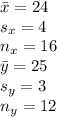
As the population standard deviations are not known, use a pooled standard deviation to estimate the standard error of the sampling distribution.

The formula of pooled standard deviation is:

`S_(p)=\sqrt{(s_(x)^(2))/(n_(x)^(2))+(s_(y)^(2))/(n_(y)^(2))}`

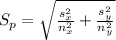
Compute the standard error for the sampling distribution as follows:

`SE=\sqrt{(s_(x)^(2))/(n_(x)^(2))+(s_(y)^(2))/(n_(y)^(2))}=\sqrt{(4^(2))/(16)+(3^(2))/(12)}=\sqrt{1+(9)/(12)}=\sqrt{(21)/(12)}=1.323`

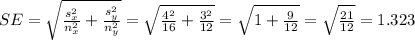
Thus, the standard error for the sampling distribution is 1.323.