Answer:
a) The explicit formula is
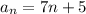
b) The 31st term of the sequence is 222
Explanation:
a) The explicit formula for an arithmetic sequence is:

The recursive formula for the sequence is
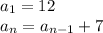
From this formula we can find the common difference by comparing to the general recursive formula:
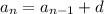
This means the common difference d=7.
We now substitute the first term
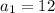 and the common difference
and the common difference
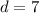 into the explicit formula:
into the explicit formula:
 to obtain:
to obtain:
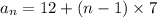
Expand to get:

Simplify:
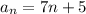
b) To find the 31st term of this sequence, we substitute n=31 in to the the explicit formula,
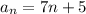 to obtain:
to obtain:
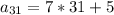
Multiply to get:

Add to get:
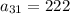
Therefore the 31st term of the sequence is 222