Question

Which statement is true of triangles QRS and MNP?

Triangle Q R S. Side Q R is 6, R S is 2, Q S is 6.32. Angle Q is 18.4 degrees, angle S is 71.6 degrees, and angle R is 90 degrees. Triangle M N P. Side M N is 3, N P is 1, M P is 3.16. Angle M is 18.4 degrees, angle P is 71.6 degrees, angle N is 90 degrees.
They are congruent because their corresponding angles are congruent and their corresponding side lengths are congruent.
They are similar because their corresponding angles are congruent and their corresponding side lengths are proportional.
They are not similar because their corresponding angles are not congruent.
They are not similar because their corresponding side lengths are not proportional.

Answer 1

They are similar because their corresponding angles are congruent and their corresponding side lengths are proportional.

Answer 2

They are similar because their corresponding angles are congruent and their corresponding side lengths are proportional.

Explanation:

we know that

If two figures are congruent, then its corresponding sides and its corresponding angles are congruent

If two figures are similar, then the ratio of its corresponding sides is proportional and its corresponding angles are congruent

In this problem

Corresponding angles are congruent

because

∠Q≅∠M

∠R≅∠N

∠S≅∠P

Corresponding sides are proportional

because

`(QR)/(MN)=(RS)/(NP)=(QS)/(MP)`

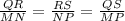
substitute the given values

`(6)/(3)=(2)/(1)=(6.32)/(3.16)`

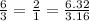
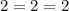`2=2=2` ----> is true

therefore

They are similar because their corresponding angles are congruent and their corresponding side lengths are proportional.