Question

Use differentials to estimate the amount of material in a closed cylindrical can that is 50 cm high and 20 cm in diameter if the metal in the top and bottom is 0.2 cm thick, and the metal in the sides is 0.05 cm thick.

Answer 1

The amount of material in a closed cylindrical is 282.6 cm³.

Explanation:

Given : A closed cylindrical can that is 50 cm high and 20 cm in diameter if the metal in the top and bottom is 0.2 cm thick, and the metal in the sides is 0.05 cm thick.

To find : Use differentials to estimate the amount of material in a closed cylindrical ?

Solution :

Let r and h be the radius and base of the cylinder.

The volume of the cylinder is V=\pi r ^2 h

Differentiate the volume,

i.e.
`dV=(\partial V)/(\partial r)\Delta r+(\partial V)/(\partial h)\Delta h`

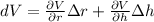
Since, the metal in the top and bottom is 0.2 cm thick, and the metal in the sides is 0.05 cm thick.

Increase in radius dr=0.05

Increase in height of cylinder is dh=0.2+0.2=0.4

Diameter d= 20 cm

Radius r=10 cm

Height h= 50 cm

Substitute the values,

`dV=(\partial (\pi r^2 h))/(\partial r)\Delta r+(\partial (\pi r^2 h))/(\partial h)\Delta h`

`dV=\pi h\cdot 2r\cdot dr+\pi r^2\cdot dh`

`dV=\pi r(2h dr+r dh)`

`dV=3.14* 10(2* 50* 0.05 +10* 0.4)`

`dV=31.4(5 +4)`

`dV=31.4(9)`

`dV=282.6`

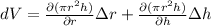
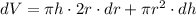
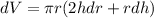
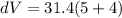
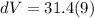
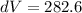
Therefore, the amount of material in a closed cylindrical is 282.6 cm³.