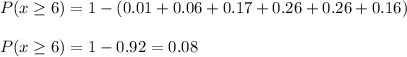Question

A company needed to downsize one department having 25 people consisting of 11 women and 14 men. Eight people were laid off, and upper management stated that the layoffs were done randomly. By chance, 44% (11/25) of the layoffs would be women. After the layoffs were made, it was found that of the eight people laid off, 6 were women. What is the probability that 6 or more women would be laid off? Which probability model should you use to calculate the answer?

Answer 1

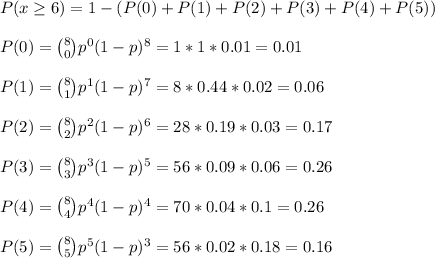
The probability that 6 or more women would be laid off is P=0.08.

Explanation:

In this case, we have a group of people and a proportion of women and men. We can think of the people laid off as a random sample taken from the population (all employees of the department).

The right model to represent that is with a binomial distribution. In this case, the sample size is n=8 and the probability of picking a woman is p=0.44.

According to this model, the probability that 6 or more women, out of 8 persons, would be laid off is:

`P(x\geq6)=1-(P(0)+P(1)+P(2)+P(3)+P(4)+P(5))\\\\ P(0)=\binom{8}{0}p^0(1-p)^8=1*1*0.01=0.01\\\\ P(1)=\binom{8}{1}p^1(1-p)^7=8*0.44*0.02=0.06\\\\ P(2)=\binom{8}{2}p^2(1-p)^6=28*0.19*0.03=0.17\\\\ P(3)=\binom{8}{3}p^3(1-p)^5=56*0.09*0.06=0.26 \\\\ P(4)=\binom{8}{4}p^4(1-p)^4=70*0.04*0.1=0.26 \\\\ P(5)=\binom{8}{5}p^5(1-p)^3=56*0.02*0.18=0.16`

`P(x\geq6)=1-(0.01+0.06+0.17+0.26+0.26+0.16)\\\\P(x\geq 6)=1-0.92=0.08`