Question

Human body temperatures have a mean of 98.20° F and a standard deviation of 0.62° F. Sally's temperature can be described by z = -1.5. What is her temperature? Round your answer to the nearest hundredth.

Answer 1

Sally's temperature is 97.27° F .

Explanation:

We are given that Human body temperatures have a mean of 98.20° F and a standard deviation of 0.62° F.

Let X = Human body temperatures

So, X ~ N(
`\mu = 98.20,\sigma^(2)=0.62^(2)`)

The z score probability distribution is given by;

Z =
`(X-\mu)/(\sigma)` ~ N(0,1)

Let Sally's temperature be x ; we are given that Sally's temperature can be described by z = -1.5; i.e.;

-1.5 =
`(x-98.20)/(0.62)`

x = 98.20 - (1.5 * 0.62)

x = 98.20 - 0.93 = 97.27° F

Therefore, Sally's temperature is 97.27° F .

Answer 2

Sally's temperature is 97.27 °F.

Explanation:

All the information given in the question tells us that the human body temperatures are normally distributed with a population's mean = 98.20°F and a standard deviation = 0.62°F.

The question gives us Sally's temperature in a z-score. We have to remember that the standard normal distribution is a particular case of a normal distribution where the mean = 0 and the standard deviation = 1.

Using the standard normal distribution, we can determine every probability associated with a normal distribution "transforming" the raw scores, coming from normally distributed data, into z-scores.

A z-score gives us the distance from the population's mean and is in standard deviation units. So, a z = 1.5 tells us that the value is 1.5 standard deviations above the mean. Conversely, a z = -1.5 tells us that the raw score is also 1.5 standard deviation from the mean, but in the opposite direction, that is, below the mean.

The formula for a z-score is as follows:

`\\ z = (x - \mu)/(\sigma)` (1)

Where

`\\ x\;is\;the\;raw\;score`.

`\\ \mu\;is\;the\;population\;mean`.

`\\ \sigma\;is\;the\;population\;standard\;deviation`.

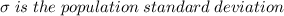Then to find x (or the raw score, that is, Sally's temperature), we need to solve the formula (1) for it to finally solve the question.

Then

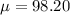`\\ \mu = 98.20^\circF` °F

`\\ \sigma = 0.62^\circF` °F

`\\ z = -1.5`

Thus (with no units)

`\\ -1.5 = (x - 98.20)/(0.62)`

`\\ (-1.5*0.62) = x - 98.20`

`\\ (-1.5*0.62) + 98.20 = x`

`\\ x = (-1.5*0.62) + 98.20`

`\\ -0.93 + 98.20`

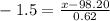
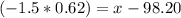
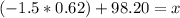
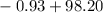
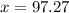`\\ x = 97.27`°F

Thus, Sally's temperature is
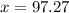`\\ x = 97.27`°F (rounding the answer to the nearest hundredth).