Answer:
When the sample size is increased from n = 9 to n = 45, the standard deviation of the sample mean decreases from 1.167 to 0.522.
Explanation:
The Central Limit Theorem estabilishes that, for a random variable X, with mean
 and standard deviation
and standard deviation
 , the sample means with size n can be approximated to a normal distribution with mean
, the sample means with size n can be approximated to a normal distribution with mean
 and standard deviation
and standard deviation
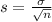
In this problem, we have that:

n = 9
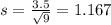
n = 45
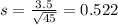
When the sample size is increased from n = 9 to n = 45, the standard deviation of the sample mean decreases from 1.167 to 0.522.