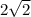Answer:
Therefore the value of c is
Explanation:
Mean Value Theorem:
It state that if f(x) is defined
(i) f(x) continuous on the interval [a,b]
(ii) f(x) differentiable on (a,b).
then there exist a one number c∈ (a,b) such that
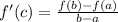
Here
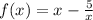
(i) f(x) is continuous on [2,4]
Since it is discontinuous at 0 and 0∉[2,4].
(ii)
 which is exist x≠0 and 0∉(2,4).
which is exist x≠0 and 0∉(2,4).
Therefore f(x) is differentiable on (2,4)
f(x) satisfies the Mean Value Theorem,
Then there exist a number c∈(2,4) such that
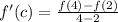
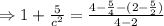
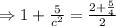
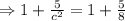
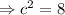
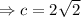
Therefore the value of c is