Answer:
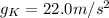
Step-by-step explanation:
From the Newton's Law of Universal Gravitation, we have that the acceleration due to gravitational force near an object of mass M, is given by:
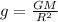
Where g is the acceleration due to gravity, G is the gravitational constant, and R is the distance between the objects.
In this case, we have the given values for planet Krypton:
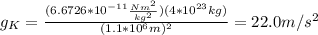
This means that the acceleration of an object in free fall near the surface of Krypton is of 22.0m/s².