Answer:
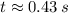
Explanation:
The vertical displacement function is
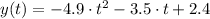 , where
, where
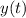 is measured in meters and
is measured in meters and
 in seconds. Ball hits the ground when
in seconds. Ball hits the ground when
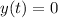 . That is:
. That is:
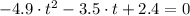
Whose roots can be found by using the General Formula for Second-Order Polynomials:

Solutions of this polynomial are:
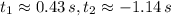
Only the first root is physically consistent.