Answer:
A triangle cannot be formed with given side lengths.
Explanation:
Given:
First side = 10.6 cm
second side = 5.6 cm
third side = 4.0 cm
We need to find whether these data can be the side lengths of the triangle.
Solution:
Now we know that;
By triangle Inequality property which states that;
"The sum of two side of the triangle must be greater than third side."
All the three condition must be fulfilled.
So checking for all three conditions we get;
1) First side + Second side > Third side
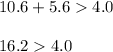
Hence this condition is True.
2) Second side + Third side > First side

Hence this condition is False.
3) Third side + First side > Second side.
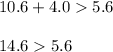
Hence this condition is True.
1 out of 3 condition is False and according to triangle property all 3 condition must be True.
Hence a triangle cannot be formed with given side lengths.