Question

Two equal forces are applied to a door. The first force is applied at the midpoint of the door; the second force is applied at the doorknob. Both forces are applied perpendicular to the door. Which force exerts the greater torque?a) the first at the midpoint.

b) the second at the doorknob.
c) both exert equal non-zero torques.
d) both exert zero torques.
e) Additional information is needed.

Answer 1

b) the second at the doorknob.

Step-by-step explanation:

The torque applied by a force is given by the equation

`\tau = F_p d`

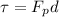
where

`\tau` is the torque

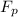`F_p` is the component of the force perpendicular to the direction between the axis of rotation and the point of application of the force

d is the arm (the distance between the axis of rotation and the point of application of the force)

In this problem, we have two equal forces F both applied perpendicular to a door, so

`F_p = F`

The first force is applied at the midpoint of the door; if we call L the width of the door, then the arm of this force is
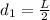`d_1=(L)/(2)`, so the torque applied by this first force is

`\tau_1 = F(L)/(2)`

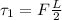
The 2nd force instead is applied at the doorknob, so the arm in this case is L:

d = L

So, the torque exerted by the second force is

`\tau_2 = FL`

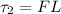
Therefore, we see that the 2nd force exerts a greater torque.