Answer:
(a) The value of x is 0.30.
(b) The probability that a reported case of rabies is not a raccoon is 0.45.
(c) The probability that a reported case of rabies is either a bat or a fox is 0.15.
Explanation:
Denote the events as follows:
R = reported case of rabies is a raccoon
F = reported case of rabies is a fox
B = reported case of rabies is a bat
O = reported case of rabies is a some other animal.
The data provided is:
P (R) = 0.55
P (F) = 0.11
P (B) = 0.04
P (O) = x.
(a)
A property of a probability distribution is that the sum of all individual properties is 1.
That is,
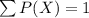
Compute the value of x as follows:
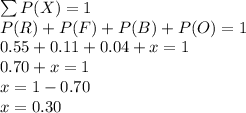
Thus, the value of x is 0.30.
(b)
The probability of the complement of an event is the probability of its not happening.
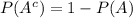
Compute the probability that a reported case of rabies is not a raccoon as follows:
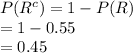
Thus, the probability that a reported case of rabies is not a raccoon is 0.45.
(c)
Compute the probability that a reported case of rabies is either a bat or a fox as follows:
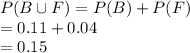
Thus, the probability that a reported case of rabies is either a bat or a fox is 0.15.