Question

In a research laboratory, a scientist measures the mass of a 1-carat diamond and finds it to be 200 milligrams, measured to the nearest hundred milligrams. The volume of the diamond is approximately 0.057 cubic centimeters. Find the density of the diamond in grams per cubic centimeter, expressing your answer to the appropriate level of accuracy. Use complete sentences to explain your reasoning.

Answer 1

3508.772mg per cm^3

Explanation:

The function for Density is Mass divided by Volume and since the question above states the mass(200mg) and the volume(0.057cm^3), finding the density of the diamond in grams per cubic centimeter should be easy.

200/0.057 = 3508.772

So the answer is that the density of the diamond is 3508.772mg per cm^3.

Answer 2

`D=3.5\ gr/cm^3`

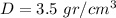
Explanation:

Density

The density of an object of mass m and volume V is given by

`\displaystyle D=(m)/(V)`

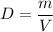
It can be expressed in common units like
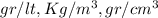`gr/lt, Kg/m^3, gr/cm^3` or any other combination of proper mass [M] by volume [V] units.

The data provided in the question is

`m=200\ mg = 0.2\ gr`

`V=0.057\ cm^3`

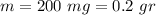
Thus, the measured density is

`\displaystyle D=(0.2\ gr)/(0.057 \ cm^3)`

`\boxed{D=3.5\ gr/cm^3}`

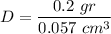
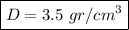
We have expressed the result with 1 decimal place because the mass was measured to the nearest hundred milligrams (or one-tenth grams). Any further decimal is senseless because that precision comes from calculations, not from measurements.