Answer:
y = -3x + 1
Explanation:
Step 1: Determine what the values of x1, x2, y1, y2 are
To find the slope use the formula, m =
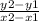
(x1, y1) is (1, -2)
(x2, y2) is (3, -8)
Step 2: Plug into the slope formula
m =
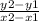
m =
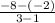
m =
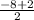
m =
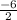
m = -3
Step 3: Determine what the values of y1, m, x1 in the point slope form
(y - y1) = m(x - x1)
(x1, y1) is (1, -2)
m = -3
Step 4: Plug into the point slope form
(y - y1) = m(x - x1)
(y - (-2)) = -3(x - 1)
y + 2 - 2 = -3x + 3 - 2
y = -3x + 1
Answer: y = -3x + 1