Answer:
B) The margin of error becomes smaller
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.
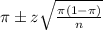
In which
z is the zscore that has a pvalue of
 .
.
The width of the confidence interval is given by:

So as n increases, the width, or margin of error, becomes smaller.
As your sample size increases (let us say from 100 to 400 cases), which of the following becomes true?
The answer is:
B) The margin of error becomes smaller