Question

Two identical conducting spheres, A and B, carry equal charge. They are separated by a distance much larger than their diameters. A third identical conducting sphere, C, is uncharged. Sphere C is first touched to A, then to B, and finally removed.

1. As a result, the electrostatic force between A and B, which was originally F, becomes ________:A. F/2B. F/4C. 3F/8D. F/16E. 0

Answer 1

C.
`(3F)/(8)`

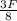
Step-by-step explanation:

Let initial charges on both spheres be,
`q`

`F=(Kq^2)/(d^2) \ \ \ \ \ \ \ \ \ \ \_i`

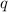
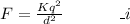
When the sphere C is touched by A, the final charges on both will be,
`(q)/(2)`

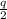
#Now, when C is touched by B, the final charges on both of them will be:

`q_c=q_d=(q/2+q)/(2)\\\\=(3q)/(4)\\`

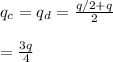
Now the force between A and B is calculated as:

`F\prime=(k*(q)/(2)* (3q)/(4))/(d^2)\\F\prime=(3F)/(8)`

Hence the electrostatic force becomes 3F/8