Answer:
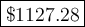
Step-by-step explanation:
For an amortized loan, paying back a fixed amount periodically, the formula for each payment is
a = P\left (\dfrac{r(1 + r)^{n}}{(1 +r)^{n} - 1}
where
a = payment amount per period
P = principal (loan amount)
r = interest rate per period
n = total number of periods (payments)
Data:
Cost = $68 000
Down payment = $20 000
Term of loan = 4 yr
APR = 6 %
Payments = monthly
Calculations:
P = $68 000 - $20 000 = $48 000
n = 4 × 12 = 48
r = 0.06/12 = 0.005
