Answer:
Fraction of the red blood cells having a surface area larger than 170
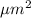 is 0.04006 or 4% .
is 0.04006 or 4% .
Explanation:
We are given that in a typical blood sample, the surface areas of the red blood cells have a normal distribution with a mean of 135
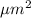 and a standard deviation of 20
and a standard deviation of 20
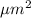 .
.
Let X = the surface areas of the red blood cells, i.e;
X ~ N(
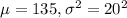 )
)
The Z score probability distribution is given by;
Z =
 ~ Standard Normal(0,1)
~ Standard Normal(0,1)
So, fraction of the red blood cells having a surface area larger than 170
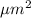 is given by P(X > 170
is given by P(X > 170
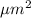 ) ;
) ;
P(X > 170) = P(
 >
>
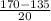 ) = P(Z > 1.75) = 1 - P(Z <= 1.75)
) = P(Z > 1.75) = 1 - P(Z <= 1.75)
= 1 - 0.95994 = 0.04006 or approx 4%.
Therefore, approx 4% of the red blood cells has a surface area larger than 170
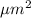 .
.