Answer: F = 211312.5ft/lb.
Step-by-step explanation: Hydrostatic pressure is pressure caused by a fluid due to the force of gravity. It is calculated following the formula:
P = ρgh.
ρ is the fluid density (62.5 lb/ft³);
g is the gravitacional acceleration( 32.2ft/s²);
h is the height of the fluid columm;
P = 62.5.32.2.h
Pressure at h = 1ft:
P = 62.5.32.2.1
P = 2012.5 psi.
Now, hydrostatic force is F =
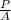
The pressure exerted on the surface is not constant. It depends on the height. So, to evaluate the force:
dF = P(h) dA
Since it a rectangular plate, dA = w.dh, where w is its width and equals 6 and dh is its height element.
That gives: dF = P(h)w.dh
 = 2012.5.6.
= 2012.5.6.
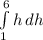 = 2012.5.6(
= 2012.5.6(
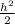 ) = 12075(
) = 12075(
 ) = 211312.5ft/lb.
) = 211312.5ft/lb.