Question

A 645-kg elevator starts from rest and reaches its cruising speed of [10] ____________________ m/s after 3.13 seconds. It moved 2.75 m during that time. a. What average power (W) is delivered by the motor during the initial acceleration of the elevator during the first 3.13 seconds?

Answer 1

Answer: v = 0.8786m/s W = 79.54J

Explanation: The blank space asks for the velocity of the elevator. Velocity is defined by the space an object moves per time it needs to travel this space. In other words: v = Δs / Δt.

According to the question, the elevator go through 2.75m in 3.13s, so velocity will be

v =
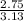`(2.75)/(3.13)` = 0.8786 m/s. This is the cruising speed.

Power is the amount of work done by an object per period of time.

W = τ / Δt

In this case, the work (τ) is due to the movement of the elevator. Using the work-energy theorem, which states that the amount of work done in a moving object can be determined by the variation of its kinetic energy, power can be rewritten as:

W = (
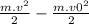`(m.v^(2) )/(2) - (m.v0^(2) )/(2)`) / Δt

As the elevator starts from rest, the initial velocity is 0.

W = (
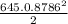`(645.0.8786^(2) )/(2)`) / 3.13

W = 79.54 J

The average power delivered by the motor is 79.54J.