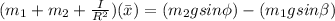Answer:
the equivalent mass :
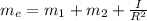
the equation of the motion of the block of mass
 in terms of its displacement is =
in terms of its displacement is =
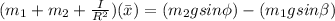
Step-by-step explanation:
Let use m₁ to represent the mass of the block and m₂ to represent the mass of the cylinder
The radius of the cylinder be = R
The distance between the center of the pulley to center of the block to be = x
Also, the angles of inclinations of the cylinder and the block with respect to the ground to be
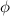 and
and
 respectively.
respectively.
The velocity of the block to be = v
The equivalent mass of the system =
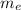
In the terms of the equivalent mass, the kinetic energy of the system can be written as:
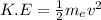 --------------- equation (1)
--------------- equation (1)
The angular velocity of the cylinder =
 : &
: &
The inertia of the cylinder about its center to be = I
The angular velocity of the cylinder can be written as:
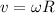
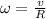
The kinetic energy of the system in terms of individual mass can be written as:
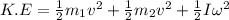
By replacing
 with
with
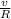 ; we have:
; we have:
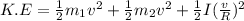
 ------------------ equation (2)
------------------ equation (2)
Equating both equation (1) and (2); we have:
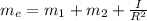
Therefore, the equivalent mass :
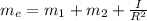 which is read as;
which is read as;
The equivalent mass is equal to the mass of the block plus the mass of the cylinder plus the inertia by the square of the radius.
The expression for the force acting on equivalent mass due to the block is as follows:

Also; The expression for the force acting on equivalent mass due to the cylinder is as follows:

Equating the above both equations; we have the equation of motion of the equivalent system to be
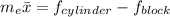
which can be written as follows from the previous derivations
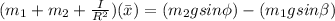
Finally; the equation of the motion of the block of mass
 in terms of its displacement is =
in terms of its displacement is =