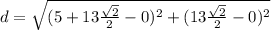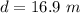Answer:
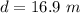
Explanation:
Let
 -----> Andrew's initial location
-----> Andrew's initial location
we know that
1) He travels 5 meters 90°north
At this moment Andrew's location is
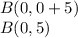
2) He moves 13 meters 45° east
At this moment Andrew's location is
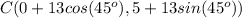
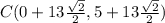
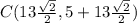
3) Find the distance between Andrew's initial location to the hidden treasure.
Find the distance between point A and point C
the formula to calculate the distance between two points is equal to
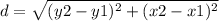
we have

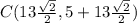
substitute the values