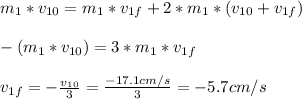Answer:
v₁f = -5.7 cm/s
Step-by-step explanation:
- Assuming no external forces acting during the collision, total momentum must be conserved, as follows:
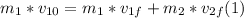
- Rearranging terms, we have:
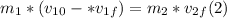
- We also know that the collision is elastic, so total kinetic energy must be conserved , as follows:
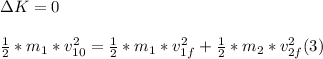
- Rearranging , and simplifying common terms, we have:
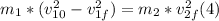
- Replacing by the givens, doing some algebra and dividing (4) by (2), we find the following relationship:
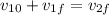
- Replacing the expression above in (1), as m₂ = 2*m₁, we can find the value of v₁f, as follows: