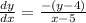Answer:
a)
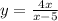
b)
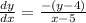
Explanation:
Step 1:-
Given 4x+5y = x y .......(1)
subtracting '5y' on both sides, we get
4x+5y-5y = x y - 5y
on simplification, we get
4x = y(x-5)
Dividing 'x-5' on both sides, we get
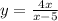
Step 2:-
by using derivative formulas

apply '

Differentiating equation (1) with respective to 'x' we get
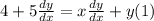
On simplification , taking common
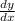 we get,
we get,