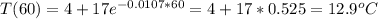Answer:
12.9 degree C
Explanation:
1 hour = 60 minutes
So the Newton's law of cooling state that the rate of changing in temperature is proportional to the different in temperature, in other words:

where t is the time in minute, k is the cooling constant and
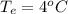 is the environmental temperature. This differential equation can be solved to have a form of
is the environmental temperature. This differential equation can be solved to have a form of
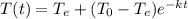
where
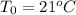 is the initial temperature.
is the initial temperature.
We know that at 25 minutes T(25) = 17
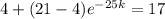

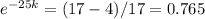
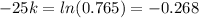

So after 60 minutes: