Option D:
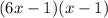 is the product of the expression
is the product of the expression
Step-by-step explanation:
The expression is
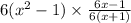
We need to determine the product of the expression.
To determine the product of the expression, we need to simplify the given expression.
Thus, we have,
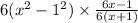
The term
 is of the form
is of the form
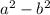
Now, we shall use the identity,
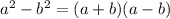
Hence, we have,
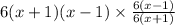
Multiplying the terms and cancelling the common terms, we get,

Hence, the product of the given expression is
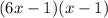
Therefore, Option D is the correct answer.