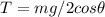Question

To carry a 100 pound cylindrical weight, two people lift on the ends of short ropes that are tied to eyelet on the top center of the cylinder. Each rope makes an angle of theta degrees with the vertical. Write the tension T of each rope as a function of Theta.

Answer 1

`\boxed {\displaystyle T=(m.g)/(2cos\theta)}`

Explanation:

Net Force

The net force exerted on an object is the sum of all vector forces applied to it. If no acceleration is achieved, the net force is zero.

We have three forces applied to the cylindrical weight: Its own weight and the two components of the tension of each rope. Knowing they form an angle
`\theta` with the vertical, then each component is given by

`T_y=Tcos\theta`

The sum of all forces in the vertical direction is

`F_y=2T_y-m.g`

The object is not accelerated, thus

`2T_y-m.g=0`

`2Tcos\theta=m.g`

Solving for T

`\boxed {\displaystyle T=(m.g)/(2cos\theta)}`

Answer 2

`T=mg/2cos \theta`

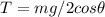
Explanation:

#Given that each rope is tied to the cylinder top and makes the same
`\angle \theta` with the vertical,:

Let tension on the rope be
`T`

#The vertical component of each T is,
`Tcos \theta`

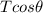
Total vertical component is
`2Tcos \theta`

We know that

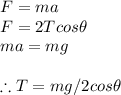`F=ma\\F=2Tcos \theta\\ma=mg\\\\\therefore T=mg/2cos \theta` #where
`g` is gravitational acceleration.

Hence the tension of each rope is
`T=mg/2cos \theta`