Question

Suppose that the useful life of a particular car battery, measured in months, decays with parameter 0.025. We are interested in the life of the battery. Find the probability that a car battery lasts more than 36 months.

Answer 1

The probability that a car battery lasts more than 36 months is 0.4066.

Explanation:

Let X = the useful life of a particular car battery.

The decay parameter is, λ = 0.025.

The random variable X follows an Exponential distribution with parameter λ = 0.025.

The probability density function of X is:

`f(x)=0.025e^(-0.025x);\ x>0`

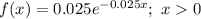
Compute the probability that a car battery lasts more than 36 months as follows:

`P(X>36)=\int\limits^(\infty)_(36) {0.025e^(-0.025x)} \, dx`

`=0.025\int\limits^(\infty)_(36) {e^(-0.025x)} \, dx`

`=0.025 |(e^(-0.025x))/(-0.025)|^(\infty)_(36)\\`

`=|-e^(-0.025x)|^(\infty)_(36)\\`

`=|-0+e^(-0.02536)|^(\infty)_(36)\\`

`=|-0+e^(-0.02536)|^(\infty)_(36)\\=0.4066`

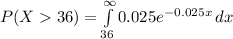
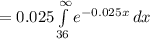
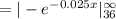
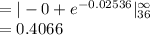
Thus, the probability that a car battery lasts more than 36 months is 0.4066.