Answer:
12 and 3.0984
Explanation:
Binomial probability distribution
Probability of exactly x sucesses on n repeated trials, with p probability.
The expected value of the binomial distribution is:
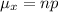
The standard deviation of the binomial distribution is:
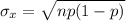
A binomial event has n = 60 trials. The probability of success for each trial is 0.20.
So we also have p = 0.2.
Then
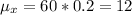
[tex]\sigma_{x} = \sqrt{60*0.2*0.8} = 3.0984
So the correct answer is
12 and 3.0984