a) 57.5 m/s
b) Yes
Step-by-step explanation:
a)
According to Faraday-Newmann-Lenz's law, the electromotive force induced in the coil due to the change in magnetic flux through it is given by:
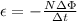
where
N is the number of turns in the coil
 is the change in magnetic flux
is the change in magnetic flux
 is the time interval
is the time interval
The change in magnetic flux can be written as
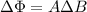
where
A is the area of the coil
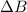 is the variation of the strength of the magnetic field
is the variation of the strength of the magnetic field
Re-writing the equation,
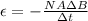
To make the bulb glowing, the induced emf must be:

And we also have:
N = 100
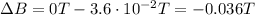

So we can find the maximum time required to induce this emf:
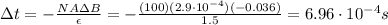
Since the length to cover in this time is
L = 4.0 cm = 0.04 m
The speed should be

b)
Yes: if the coil is moved at a speed of 57.7 m/s, then the potential difference induced in the bulb will be 1.5 V, which is enough to make the bulb glowing.