Question

A 3.92 cm tall object is placed in 31.3 cm in front of a convex mirror. The focal

length is 12.7 cm. What is the magnification of the image?

Answer 1

0.29

Step-by-step explanation:

We can find the position of the image by using the mirror equation:

`(1)/(f)=(1)/(p)+(1)/(q)`

where:

f is the focal length of the mirror

p is the distance of the object from the mirror

q is the distance of the image from the mirror

For the mirror in this problem:

f = -12.7 cm (the focal length of a convex mirror is negative)

p = 31.3 cm (distance of the object)

Solving for q, we find the position of the image:

`(1)/(q)=(1)/(f)-(1)/(p)=(1)/(-12.7)-(1)/(31.3)=-0.111 cm^(-1)\\q=(1)/(-0.111)=-9.1 cm`

And so, the magnification of the image is:

`M=-(q)/(p)`

And substituting,

`M=-(-9.1)/(31.3)=0.29`

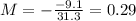
Which means that the image is upright (positive sign) and diminished (M is smaller than 1).