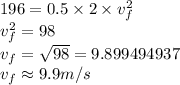Question

8. A toy car with a mass of 2 kg starts at rest. A spring performs 196 joules of

work on the car.
A. What was the change in kinetic energy? Why?
B. What was the initial kinetic energy of the car? Why?
C. What was the final kinetic energy of the car? Why?
E. What is the toy car’s final velocity?

Answer 1

(a) 196 J

(b) 0

(c) 196 J

(d) 9.9 m/s

Step-by-step explanation:

(a)

Change in kinetic energy is given by subtracting final kinetic energy from the initial kinetic energy. In this case, the initial kinetic energy is zero since it's at rest and as we know, kinetic energy is given by
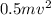`0.5mv^(2)` where m is the mass of the toy car and v is the velocity.

The final kinetic energy is what the spring posses hence 196 J. Substituting 2 Kg for m and 0 m/s for initial velocity since it's at rest then

Change in kinetic energy=
`0.5m(v_f^(2)-v_i^(2))= 196 J- (0.5* 2 kg * 0^(2))`

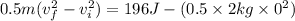
Therefore, change in kinetic energy is equivalent to 196 J

(b)

As already shown in part a above, kinetic energy is given by
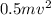`0.5mv^(2)` and since the toy car was initially at rest, then the initial velocity is zero. Substituting 2 Kg for m and 0 m/s for initial velocity since it's at rest then the initial kinetic energy will be

`KE_i= 0.5* 2* 0^(2)=0 J`

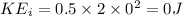
Therefore, the initial kinetic energy is zero.

(c)

Since the spring performs work equivalent to 196 J, this is the final kinetic energy of the toy car hence the answer is 196 J

(d)

The final kinetic energy is also given by
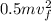`0.5mv_f^(2)` and in this case we have the value of final kinetic energy hence we can substitute 2 Kg for mass to get the velocity

`196=0.5* 2* v_f^(2)\\v_f^(2)=98\\v_f=√(98)=9.899494937\\v_f\approx 9.9 m/s`