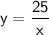Question

NO LINKS!! Explain your answer (show the support by showing the changes in x and y on your table). If the relationship is linear, inverse or exponential, write the equation. #9

​

Answer 1

Linear relationship: increasing or decreasing one variable will cause a corresponding increase or decrease in the other variable.

Inverse relationship: the value of one variable decreases as the value of the other variable increases.

Exponential relationship: a constant change in the independent variable (x) gives the same proportional change in the dependent variable (y)

Question 5

As the x-value increases (by one unit), the y-value decreases.

Therefore, this is an inverse relationship.

The y-values can be calculated by dividing 25 by the x-value.

`\sf y=(25)/(x)`

Answer 2

• relationship: inverse
• equation: y = 25/x

Explanation:

You are correct that the relationship is inverse, and that the product of x and y is a constant, 25.

__

The equation should be written so that y is a function of x:

`\boxed{y=(25)/(x)}`

The x in the denominator clearly shows that y is proportional to the inverse of x.