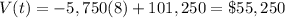Answer:
Part A)
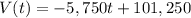
Part B)
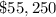
Explanation:
Part A) Express the value of the bulldozer, V, as a function of how many years old it is, t.
Let
V ----> the value of the bulldozer (dependent variable or output value)
t ----> the number of years (independent variable or input value)
we know that
The linear function in slope intercept form is equal to
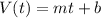
we have the ordered pairs
(0,101,250) and (15,15,000)
Find the slope
The formula to calculate the slope between two points is equal to
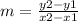
substitute
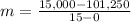
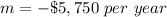 ----> is negative because is a decreasing function
----> is negative because is a decreasing function
The value of b is the initial value
so
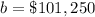
substitute
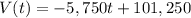
Part B) The value of the bulldozer after 8 years is
For t=8 years
substitute the value of t in the linear equation