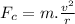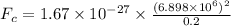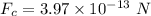Question

A beam of protons moves in a circle of radius 0.20 m. The protons move perpendicular to a 0.36-T magnetic field. (a) What is the speed of each proton? (b) Determine the magnitude of the centripetal force that acts on each proton.

Answer 1

a)
`v=6.898* 10^(6)\ m.s^(-1)` is the speed of each proton

b)
`F_c=3.97* 10^(-13)\ N`

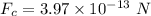
Step-by-step explanation:

Given:

radius of path of motion,
`r=0.2\ m`

we know charge on protons,
`q=1.6* 10^(-19)\ C`

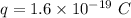
magnetic field strength,
`B=0.36\ T`

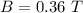
we've mass of proton,
`m=1.67* 10^(-27)\ kg`

a)

From the equivalence of magnetic force and the centripetal force on the proton:

`F_B=F_C`

`q.v.B=(m.v^2)/(r)`

`q.B=(m.v)/(r)`

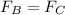
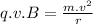
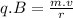
where:

v = speed of the proton

`(1.6* 10^(-19))* 0.36=(1.67* 10^(-27)* v)/(0.2)`

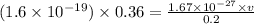
`v=6.898* 10^(6)\ m.s^(-1)` is the speed of each proton

b)

Now the centripetal force on each proton:

`F_c=m.(v^2)/(r)`

`F_c=1.67* 10^(-27)* ((6.898* 10^6)^2)/(0.2)`

`F_c=3.97* 10^(-13)\ N`