Answer:
Critical value for this hypothesis test is 6.571
Explanation:
We are given that a random sample of 15 students was selected and the sample standard deviation for the time needed to complete the exam was found to be 4.0 minutes.
A professor would like to test the hypothesis that the average number of minutes that a student needs to complete a statistics exam has a standard deviation that is less than 5.0 minutes.
So, Null Hypothesis,
 :
:
 = 5.0 minutes
= 5.0 minutes
Alternate Hypothesis,
 :
:
 < 5.0 minutes
< 5.0 minutes
Now, the test statistics used here for testing population standard deviation is;
T.S. =
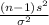 ~
~

where, s = sample standard deviation = 4.0 minutes
n = sample size = 15
So, test statistics =
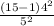 = 8.96
= 8.96
At, 5% level of significance the chi-square table gives critical value of 6.571 at 14 degree of freedom. Since our test statistics is more than the critical value as 8.96 > 6.571 so we have insufficient evidence to reject null hypothesis.