Question

The driving distance for the top 100 golfers on the PGA tour is between 284.7 and 310.6 yards. Assume that the driving distance for these golfers is uniformly distributed over this interval. What is the probability the driving distance for one of these golfers is more than 300 yards

Answer 1

`X \sim Unif(a=284.7, b = 310.6)`

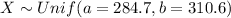
And the density function is given by:

`f(x) = (1)/(b-a)= (1)/(310.6-284.7)= 0.0386, 284.7 \leq x \leq 310.6`

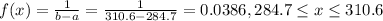
And the cumulative distribution function is given by:

`F(X) = (x-a)/(b-a)= (x-284.7)/(25.9)`

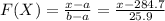
And we want the following probability:

`P(X>300)`

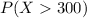
And we can use the complement rule and we got:

`P(X>300)= 1-P(X<300) = 1-F(300) = 1-(300-284.7)/(25.9)= 1-0.5907= 0.4093`

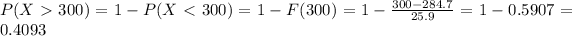
Explanation:

Let X the random variable that represent the driving distance and we know that the distribution for X is given by:

`X \sim Unif(a=284.7, b = 310.6)`

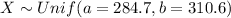
And the density function is given by:

`f(x) = (1)/(b-a)= (1)/(310.6-284.7)= 0.0386, 284.7 \leq x \leq 310.6`

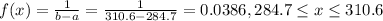
And the cumulative distribution function is given by:

`F(X) = (x-a)/(b-a)= (x-284.7)/(25.9)`

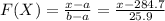
And we want the following probability:

`P(X>300)`

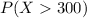
And we can use the complement rule and we got:

`P(X>300)= 1-P(X<300) = 1-F(300) = 1-(300-284.7)/(25.9)= 1-0.5907= 0.4093`

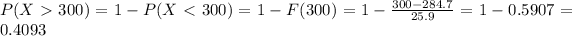
And that would be the final answer for this case.