Question

A chemist fills a reaction vessel with 7.92 atm nitrogen (N2) gas, 2.02 atm hydrogen (H2) gas, and 2.11 atm ammonia (NH3) gas at a temperature of 25.0°C

Under these conditions, calculate the reaction free energy ΔG for the following chemical reaction

N2(8) +3H2 2NH3 (g)

Use the thermodynamic information in the ALEKS Data tab. Round your answer to the nearest kilojoule.

Answer 1

Answer: The Gibbs free energy of the given reaction is -40 kJ

Step-by-step explanation:

The given chemical equation follows:

`N_2(g)+3H_2(g)\rightarrow 2NH_3(g)`

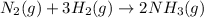
The equation for the standard Gibbs free change of the above reaction is:

`\Delta G^o_(rxn)=[(2* \Delta G^o_f_((NH_3(g))))]-[(1* \Delta G^o_f_((N_2(g))))+(3* \Delta G^o_f_((H_2(g))))]`

We are given:

`\Delta G^o_f_((NH_3(l)))=-16.45kJ/mol\\\Delta G^o_f_((H_2(g)))=0kJ/mol\\\Delta G^o_f_((N_2(g)))=0kJ/mol`

Putting values in above equation, we get:

`\Delta G^o_(rxn)=[(2* (-16.45))]-[(1* (0))+(3* (0))]\\\\\Delta G^o_(rxn)=-32.9kJ/mol`

The equation used to Gibbs free energy of the reaction follows:

`\Delta G=\Delta G^o+RT\ln Q_(eq)`

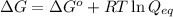
where,

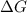`\Delta G` = free energy of the reaction

`\Delta G^o` = standard Gibbs free energy = -32.9 kJ/mol = -32900 J/mol (Conversion factor: 1 kJ = 1000 J)

R = Gas constant = 8.314J/K mol

T = Temperature =
`25^oC=[273+25]K=298K`

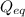`Q_(eq)` = Ratio of concentration of products and reactants at any time =
`((p_(NH_3))^2)/((p_(H_2))^3* p_(N_2))`

`p_(NH_3)=2.11atm`

`p_(N_2)=7.92atm`

`p_(H_2)=2.02atm`

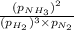
Putting values in above equation, we get:

`\Delta G=-32900J/mol+(8.314J/K.mol* 298K* \ln (((2.11)^2)/((2.02)^3* 7.92)))\\\\\Delta G=-39553.04J/mol=--39.55kJ=-40kJ`

Hence, the Gibbs free energy of the given reaction is -40 kJ